Suma de funciones
(f + g)(x) = f(x) + g(x)
Dominio
D(f + g) = D f  D g
D g
 D g
D gEjemplo


Df =  − {2}Dg = [0, ∞)
− {2}Dg = [0, ∞)
 − {2}Dg = [0, ∞)
− {2}Dg = [0, ∞)
D(f + g) = [0, 2)  (2, ∞)
(2, ∞)
 (2, ∞)
(2, ∞)Propiedades
Asociativa:
f(x) + [g(x) + h(x)] = [f(x) + g(x)] + h(x)
Conmutativa:
f(x) + g(x) = g(x) + f(x)
Elemento neutro:
La función constante: f(x) = 0.
Elemento simétrico:
La función opuesta: −f(x).
Resta de funciones
(f − g)(x) = f(x) − g(x)
Dominio
D(f − g) = D f  D g
D g
 D g
D g
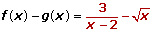
D(f + g) = [0, 2)  (2, ∞)
(2, ∞)
 (2, ∞)
(2, ∞)Producto de funciones
(f · g)(x) = f(x) · g(x)
Dominio
D(f · g) = D f  D g
D g
 D g
D g
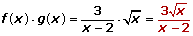
D(f + g) = [0, 2)  (2, ∞)
(2, ∞)
 (2, ∞)
(2, ∞)Propiedades
Asociativa:
f(x) · [g(x) · h(x)] = [f(x) · g(x)] · h(x)
Conmutativa:
f(x) · g(x) = g(x) · f(x)
Elemento neutro:
La función constante: f(x) = 1.
Distributiva:
f(x) · [g(x) + h(x)] = [f(x) · g(x)] + [f(x) · h(x)]
División de funciones
(f / g)(x) = f(x) / g(x)
Dominio
D(f + g) =(D f  D g) − {x
D g) − {x 
 / g(x) = 0}
/ g(x) = 0}
 / g(x) = 0}
/ g(x) = 0}

D f =  − {2}D g = [0, ∞) g(x) ≠ 0
− {2}D g = [0, ∞) g(x) ≠ 0
 − {2}D g = [0, ∞) g(x) ≠ 0
− {2}D g = [0, ∞) g(x) ≠ 0
D(f + g) = (0, 2)  (2, ∞)
(2, ∞)
http://www.ditutor.com/funciones/operaciones_funciones.html
 (2, ∞)
(2, ∞)http://www.ditutor.com/funciones/operaciones_funciones.html
No hay comentarios:
Publicar un comentario